Due to the hidden nature of bipolar disorder and the challenges of combating it when noticed late, this paper investigate the prevalence build up of unnoticeable and unreported mood disorder within the staff and students in selected tertiary institution using validated mood disorder questionnaire. The study used the multivariate mutual information matrix equivalent of the covariance matrix to obtain the nonlinear mutual information density from which the graphical model behavior of mood disorder in males and females based on age can be explored. The inclusion criteria include participant consent along with ensuring that the participant has not reported for treatment at any psychiatric facility. A total of twenty thousands (20000) mood disorder questionnaires were distributed within the south west and south-south part of Nigeria. Out of the 98% and 95% of the females (6212) and males (6276) respectively that have the tendency of being at the risk of suffering from various known conditions of bipolar disorders, the graphical model reveals that almost 40% of the females experience noticeable mood swing at two different periods between age 18-26 and 31-38 while about 70% male counterpart experience sharp mood swing between ages 35-42. This may not be unconnected to academic, emotional, marriage, job and family pressure; hence a routine mood swing check to facilitate early recognition of mood disorder will prevent future bipolar problems.

Keywords: mood disorder, gender, age, nonlinear, mutual information, multivariate normal

Though everyone has mood swings that are beyond normal from time to time, it is only when these moods becomes extreme and interfere with personal and professional life that bipolar disorder may be present and a psychiatric assessment may be warranted. This rare experience of abnormal mood swings explained the silent nature of the bipolar disease that makes it linger for a long time without been noticed or reported.¹ However, if reported late, the severity of the disease becomes pronounced and difficult to treat. Such individual are prone to violence and thus may be dangerous to people around him or her. So early recognition and effective early treatment is vital to the future well being of people with bipolar mood disorder.²

Hence this study used the validated mood disorder questionnaire to: 

1. Determine the prevalence of unnoticed and unreported mood disorder build up between staffs and students of selected tertiary institutions in Nigeria.
2. Comparing the trend of mood disorder behaviour between males and females with respect to ages using multivariate nonlinear mutual information density.

Starting with review of few results in multivariate mutual information, we introduce the mutual information matrix equivalent of covariance matrix into the multivariate normal density.

Definition 1: The mutual information between two random vectors X and Y is

$I\left(X,Y\right)=H\left(X\right)+H\left(Y\right)-H\left(X,Y\right)$    (1); this is analogous to $X{\displaystyle \cup Y}$  expansion. 

Definition 2: The mutual information among three random vectors X, Y and Z is $I\left(X,Y,Z\right)=H\left(X\right)+H\left(Y\right)+H\left(Z\right)-H\left(X,Y\right)-H\left(X,Z\right)-H\left(Y,Z\right)+H\left(X,Y,Z\right)$   (2).³ Also $I\left(X,Y,Z\right)$  is analogous to the set expansion $X{\displaystyle \cup Y}{\displaystyle \cup Z}$ .

Definition 3: Recall that covariance matrix $\Sigma =\left[\begin{array}{cc}{\Sigma }_{xx}& {\Sigma }_{xy}\\ {\Sigma }_{xy}& {\Sigma }_{yy}\end{array}\right]$ then the relationship between  $I\left(X,Y\right)$  and $\Sigma$ can be expressed as $I\left(X,Y\right)\propto \log \left[{\Sigma }_{xx}{\Sigma }_{yy}\right]+\log \left[1-{\rho }^2\right]$  (3).⁴

Next, we substitute the mutual Information $I\left(X,Y\right)$  matrix equivalent of covariance structure $\Sigma$  into the multivariate normal distribution see Lindsey⁵ to derive the joint density.

Hence $f(x,y)=\frac{1}{2\pi }\frac{{\exp }^{\frac{-1}{2}\left[e^{{}^{\frac{1}{2}H\left(X,Y\right)-H\left(X\right)}}{\left(x-{\mu }_x\right)}^2+e^{{}^{\frac{1}{2}H\left(X,Y\right)-H\left(Y\right)}}{\left(y-{\mu }_y\right)}^2\right]}}{{\left|e^{I\left(X,Y\right)}\right|}^{\frac{1}{2}}}$  (4)

Also substituting (2), we obtain the joint density of vectors (X,Y,Z ) as

$f\left(x,y,z\right)=\frac{1}{{\left(2\pi \right)}^{3/2}}\frac{{\exp }^{\frac{-1}{2}\left[K_1{\left(x-{\mu }_x\right)}^2+K_2{\left(y-{\mu }_y\right)}^2+K_3{\left(Z-{\mu }_z\right)}^2\right]}}{{\left|e^{I\left(X,Y,Z\right)}\right|}^{\frac{1}{2}}}$ (5)

respectively.

Where $K_1=e^{H\left(X,Y\right)+H\left(X,Z\right)-H\left(Y\right)-H\left(Z\right)-\frac{2}{3}H\left(X,Y,Z\right)}$ ; $K_2=e^{H\left(X,Y\right)+H\left(Y,Z\right)-H\left(X\right)-H\left(Z\right)-\frac{2}{3}H\left(X,Y,Z\right)}$ ; and $K_3=e^{H\left(X,Z\right)+H\left(Y,Z\right)-H\left(X\right)-H\left(Y\right)-\frac{2}{3}H\left(X,Y,Z\right)}$ .  See Shanon,⁶ Tho Pharm et al.,⁴ Reginald^7,8 for mathematical expectation and properties of entropies [ $H(.)$ ].

Prevalence of mood disorder

Question 1: Has there ever been a period of time when you were not your usual self (while not on drugs or alcohol) and you acted irrational to any of the following questions.

Question 2:  If you checked YES to more than one of the above ‘questions 1’, have several of these ever happened during the same period of time? Do you express irrational behavior often times (irrational habits) as a show of your displeasure or acceptance of a situation.

Question 3: How much of a problem did any of these cause you -- like being unable to work; having family, money, or legal troubles; or getting into arguments or fights? That is has your irrational behavior or irrational habit landed you in any problem.

Estimation of entropies and mutual information from table 4

H(X) = 0.861303, H(Y) = 0.693133, H(Z) = 1.089852, H(X,Y) = 1.552329, H(X,Z) = 1.933412, H(Y,Z) = 1.763359, H(X,Y,Z) = 2.60355802, I(X,Y) = 0.0021, I(X,Z) = 0.02, I(Y,Z) = 0.02, I(X,Y,Z) = -0.001258, ${\Sigma }_{xx}=0.343434$ , ${\Sigma }_{yy}=0$ , ${\Sigma }_{zz}=0.63227159$ ;  ${\Sigma }_{xy}=0$ ; ${\Sigma }_{xz}=0.082225248$ ${\Sigma }_{yz}=0$ .

Female Partition: H(X) = 0.7641, H(Z) = 0.8668, H(X,Z) = 1.2768, ${\Sigma }_{xx}=1.1807$ ,  ${\Sigma }_{zz}=0.8696$ , ${\Sigma }_{xz}=2.0976$ .

Male Partition: H(X) = 0.7882, H(Z) = 0.8966, H(X,Z) = 1.3268, ${\Sigma }_{xx}=1.2081$ ,  ${\Sigma }_{zz}=1.0333$ , ${\Sigma }_{xz}=2.5303$

Hence the multivariate nonlinear dependence measure for mood disorder (X), gender (Y) and age (Z) has the density $f\left(X,Y,Z\right)=e^{-\frac{1}{2}\left[0.623{(X-{\mu }_X)}^2-0.164\left(X-{\mu }_X\right)\left(Z-{\mu }_Z\right)-0.868{\left(Z-{\mu }_Z\right)}^2+0.689{\left(Y-1\right)}^2\right]}$  (6).

However, studying the partition of female and male mood disorder behaviour based on age, the quadratic form of exponent (6) is
$-0.04676{(X-{\mu }_X)}^2-4.1952\left(X-{\mu }_X\right)\left(Z-{\mu }_Z\right)+0.387{\left(Z-{\mu }_Z\right)}^2$
 and $-0.0752{(X-{\mu }_X)}^2-5.0606\left(X-{\mu }_X\right)\left(Z-{\mu }_Z\right)+0.19226{\left(Z-{\mu }_Z\right)}^2$  for female and male respectively.

Next we investigate the graphical relationship between mood disorder and age based on gender.

Out of 23,100 questionnaires giving out to participants that shows interest, only 16,000 considered of those recovered and filled appropriately were used in the study. The female and male participants in the study are of equal proportion. A total of 12488 (78%) of the respondents agreed that they have had reasons to act irrational in the last one month despite not been under the influence of alcohol or drug. Six thousand two hundred and twelve of the respondents that agreed to irrational behaviour are females; the remaining 50.2% are males (6276).  Out of the six thousand two hundred and twelve (6212) females, four thousand four hundred and sixty nine (72%) agreed that their irrational behaviour has landed them in problems ranging from minor (38%), moderate (26%) to serious problems (8%). Likewise of the six thousand two hundred and seventy six males that behaved irrational; five thousand one hundred and seventy one of them (82%) has landed in problem as a result of their irrational behavior. The study further reveals that 98% and 95% of the females (6212) and males (6276) respectively have the tendency of being at the risk of suffering from bipolar I and II disorders without noticing since it’s not symptomatic at initial stage (Table 1-4).

The prevalence of bipolar mood disorder build up in males and females has a high level of resemblance (Figure 1&2). The majority of the respondents both males and females has mood disorder score that ranges between 6 and 7. Though more females appears to have a mood score of between 7 and 8 compared to their male counterpart, the population percentage of males with mood score from 8 above is higher than that of females.

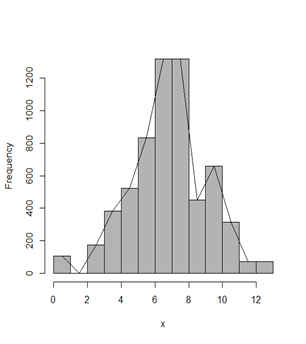
Figure 1 Prevalence (frequency) of mood disorder build up in females.

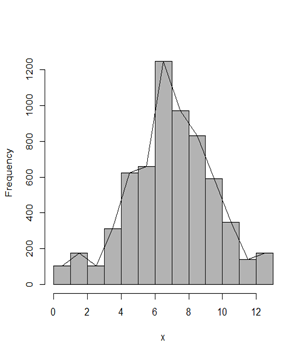
Figure 2 Prevalence (frequency) of mood disorder build up in male.

Figure 3 compares the multivariate nonlinear density of the mood disorder model in female and male within the age of 18-47 years. The curve reveals that females’ experiences high noticeable mood disorder scores due to irrational behaviour twice within the age of 18-47 years with the first and second mood swing occurring within the age bracket 18-26 years and 31-38 years respectively. However within the age of 27-30 years and 40-47 years, the mood swing of female is relatively mild. The first swing may be due to emotional struggle due to external influence like peer pressure, opposite sex, indecision, uncertainty about the next line of action and so on while marriage pressure, job pressure and other external uncontrollable influence may be the likely cause of the second swing. Despite the sharp swing periods, the period of mild swing between the ages 27-30, may not be unconnected to just getting employment, job satisfaction, home satisfaction and so on while the mild controllable swing beyond the age of 40, is likely to be due to ageing and submission totally to faith of what will be will be.  Unlike the females, the males’ mood swings were relatively mild over a long age range of between 18-34 years with a slight noticeable increase in mood disorder between the age of 25 and 27 years. The slight mood disorder may be as a result of lack of job employment coupled with stagnancy as regards the next line of progress. However, beyond the age of 34 years, the male mood disorder model reveals that the males experience sharp noticeable mood disorder increase that lingers between the ages of 35- 42 years and has its maximum likelihood value around 0.7 indicating that more than 70% of the male experience mood swing within this period. The mood swing may be due to family pressure, lack of good profitable job, marriage demand, and so on.

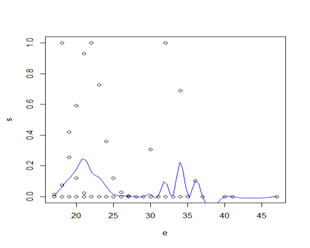
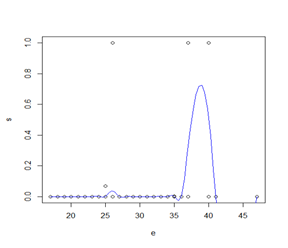
Figure 3 Comparing mood disorder behaviour in female and male at different ages.

Besides the mood disorder discrepancy between genders, figure 4 reveals the overall mood disorder behaviour of respondents against their ages. The mood swing of respondents’ experience a build up as the ages of the respondents’ increases from 18-23 years among 40% of the population within that age bracket; this is likely due to emotional and academic struggles since most respondents within this age bracket are students. Between the age of 25years and 27years, a noticeable mood disorder increase to a level of about 66% was observed. Since within the age of 25-27 years we have a mixture of respondents who are both students and staffs, then the sharp increase in mood disorder may be due to long time of academic struggle beyond the required time, the uncertainty of good job employment after school, job pressure of those employed and so on. A more significant mood disorder increase covering about 90% of the population was noticeable between the ages of 28-35 years. This may be due to lack of reliable suitors mostly for the females and possibly lack of children for those females that are already married couple with low financial status of the males. Beyond the age of 35 years, the mood swing dropped a little bit to a value of 75% and further to a value 54% around the age of 40 years. However beyond the age of 40 years, the mood swing reveals a decline to a value of about 33% and finally to almost zero value; this may be due to ageing effect of allowing things to go according to faith.

Figure 4 Overall mood disorder behaviour of students and staffs against age.

Though some prevalence differences exist between females and males as regards their mood disorder tendency on face value; a deep study of the model behavior reveals that the discrepancy is age dependent. The study recommends that parents of female students are advised to be watchful of their wards emotional balance when they are between the age brackets of 18-26. They are however not to be left alone between the age of 31-38 even in marriage. There emotional and psychological struggles should be shared and addressed to prevent unnecessary mood disorder build up. Also family dependants should also reduce the pressure they put on men whose age is above 34 years most especially those who are working and are likely not married or are facing external and internal psychological challenges.

The participants in the study are adolescence and early adults. So the late adults are not captured.

None.

Author declares that there are no conflicts of interest.

1. www.blackdog.
2. www.health.gov.au/mentalhealth.
3. Sunil Srinivasa. Review on Multivariate Mutual Information. 2003
4. Pham TH, Bao Ho T, Nguyen QD, et al. Multivariate Mutual Information measures for Discovering Biological Networks. 2012.
5. Lindsey JK. Multivariate Elliptically Contoured Distributions for Repeated Measurements. Biometrics. 1999;55(4):1277–1280.  
6. Shanon CE. A mathematical theory of communication. Bell sys Tech J. 2001;27(3):379–423.
7. Reginald Smith. A mutual information approach to calculating non linearity. The ISI journal for rapid dissemination of statistical research ArXiv. 2015;1–13.
8. Arellano-valley RB, Contreras-reyes JE, Genton MG. Shanon Entropy and mutual information for multivariate and skew-elliptical distribution. Scand J Statist. 2012;43–62.